How to Properly Convert Percent to Decimal: A Simple Guide for 2025

Understanding how to **convert percent to decimal** is a fundamental skill in mathematics that you will use in many aspects of everyday life. Whether you’re calculating percentages in finance, statistics, or general problem-solving, knowing the **percent to decimal method** will help you make sense of numerical data effectively. In this guide, we will explore simple techniques and examples of **percent to decimal** conversion, ensuring you grasp the necessary concepts for accurate calculations.

Understanding Decimal Conversion

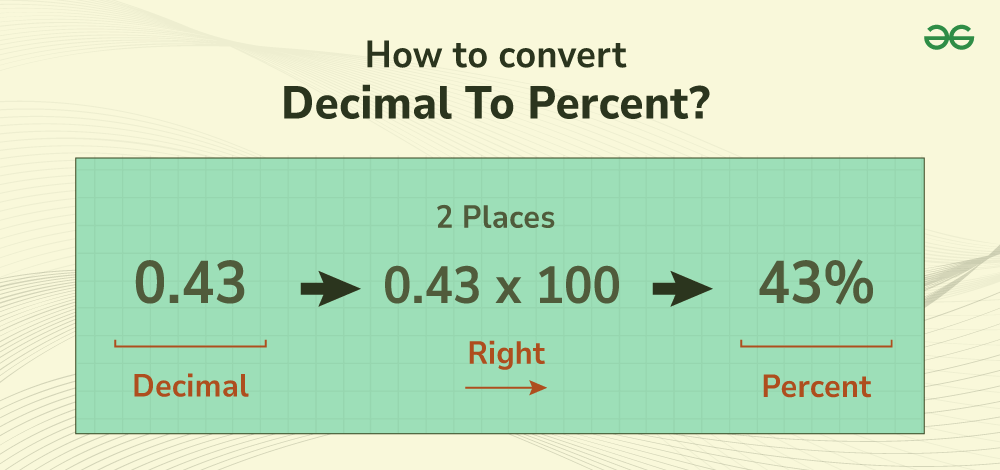
The **decimal representation of percent** is essential in many areas, from academics to real-life applications. The **percent notation in decimal** helps to clarify how much of a whole is being represented. To convert a **percentage to decimal**, you essentially move the decimal point two places to the left. For example, to change 25% into a decimal, you move the decimal point from the right side of the number to the left, resulting in 0.25. This basic understanding simplifies the **calculation of decimal from percent** and is foundational for various **percentage calculations** you may encounter.

Percent Decimal Formula

To accurately convert a **percent to decimal**, you can use the **percent decimal formula**: simply divide the percentage by 100. This means that if you have 75%, you would calculate it as follows: 75 ÷ 100 = 0.75. This formula not only reinforces the idea of moving the decimal but also allows for immediate applications in financial and statistical calculations, rendering it a vital part of your mathematical toolkit.

Examples of Percent to Decimal Conversion

Let’s explore a few **examples of percent to decimal** conversion to solidify your understanding. Imagine you need to convert 50% into a decimal. Following the formula, you would take 50 ÷ 100, equating to 0.50. If you’re dealing with percentages that are less common, such as 7.5%, the same principle applies: 7.5 ÷ 100 = 0.075. These straightforward techniques will enhance your skills in **converting percentages to decimals**, which is valuable in various math contexts.

Methods for Simple Percent Conversion

There are numerous strategies to ensure quick and accurate **simple percent conversion**. One commonly used method is employing calculators or tools designed for **decimal conversion**. However, understanding how to perform conversions manually is equally important, as it strengthens your mathematical aptitude and reinforces theories that you may encounter in more advanced **mathematical properties**.

Quick Conversion Techniques

In addition to calculators, memorizing key percentages can greatly aid in quick conversions. For example, knowing that 10% is equivalent to 0.1 and 20% equals 0.2 can speed up your calculations. Practicing with standard ratios enhances your agility with numbers and allows you to apply these techniques efficiently in **financial calculations** or **everyday math skills** where quick calculations are often required.

Teaching Decimals Effectively

If you’re looking to teach the concept of **understanding decimal conversion**, visual aids and graphics can substantially boost comprehension. Use charts that outline how different percentages compare to their decimal equivalents, or even practical, relatable examples such as calculating discounts. These teaching methods not only lay a solid foundation in **mathematics** but also enhance learners’ ability to grasp subsequent concepts easily, emboldening their proficiency with numbers.

The Importance of Decimal Calculations in Finance

In finance, knowing how to easily **convert percentages to decimals** plays a crucial role in understanding interest rates, loan terms, and investment returns. For instance, if you have an investment that increases by 10%, interpreting this as 0.10 in decimal format can lead to correct computations for your total returns. **Learning percentages** and their respective decimal forms not only impacts personal finance decisions but also enhances overall **financial literacy**, paving the way for better money management.

Real-world Financial Examples

Imagine you want to calculate a 15% tip on a $50 meal. To apply the **percent to decimal method**, first convert the 15% into 0.15. Then simply multiply: $50 × 0.15 = $7.50. Comprehending this conversion is key to effective financial decision-making, proving once again the significance of understanding **decimal conversion** in everyday scenarios.

Common Errors in Percent to Decimal Conversion

Many people make mistakes while converting percentages, often because of improper placement of the decimal or forgetting to divide by 100. For instance, 150% might mistakenly be read as 1.5 instead of 1.50. Recognizing these common pitfalls will help improve your conversion accuracy. Always double-check your calculations to ensure that your understanding and execution of the **percentage to decimal rule** are precise.

Key Takeaways

• To convert percent to decimal, move the decimal point two places to the left or divide by 100.
• Memorizing key percentages can act as shortcuts in various calculations.
• Practical applications in finance illuminate the relevance of this conversion skill.
• Understanding decimal conversion strengthens foundational math skills essential for advanced mathematical applications.

FAQ

1. What is the easiest method to convert percent to decimal?

The easiest way to **convert percent to decimal** is by moving the decimal point two places to the left or dividing the percentage value by 100. For instance, to convert 85%, you move the decimal to get 0.85.

2. Can you provide a formula for converting percentages?

Yes, the **percent decimal formula** is simple: Decimal Value = Percentage ÷ 100. This formula helps you accurately change percent to decimal and apply it across various math problems.

3. How does converting percentages benefit financial calculations?

Understanding how to **convert percentages** into decimals is crucial in finance, as it allows for accurate assessments of interest rates, returns on investments, and budgeting. It simplifies complex calculations into manageable figures.

4. Are there resources available for learners struggling with decimal conversions?

Absolutely! There are numerous **educational resources** online that offer interactive tools, tutorials, and practice exercises that aim to enhance students’ understanding of **decimal conversions** and facilitate easier learning.

5. What role does decimal representation play in statistics?

In statistics, **decimal representation of percent** facilitates informed data analysis. Properly interpreting percentages in decimal form allows for precise statistical calculations and clearer presentation of numerical data.

6. How can visual tools aid in teaching decimal conversion?

Visual tools like charts and diagrams vividly illustrate the relationship between percentages and decimals, making complex math topics more relatable and easier to comprehend for learners of all ages.

7. What should I do if I make an error in conversion?

If an error occurs during **step-by-step conversion**, it’s vital to revisit and examine your calculations. Double-checking intentions and confirming results ensures your foundation remains intact in both practical and academic situations.